CBSE Class 12 Maths Exam 2024 Important Questions: The Central Board of Secondary Education is the largest and one of the most famed school boards in India, and lakhs of students are currently enrolled in it. The CBSE conducts the Class 12 board exams annually. The next paper is arguably the most important for science and commerce stream students: Maths on 9 March. Maths is essential for non-medical science and commerce aspirants and is also required in subjects like physics, statistics and accounts. CBSE Class 12 Maths requires extensive practice, especially important topics like Calculus and Algebra. There will be five sections in the 2024 CBSE Class 12 Maths exam, and the last section E will comprise two case study-based questions of 4 marks. These questions are quite important from the exam point of view, and you can check and practice the solved versions here.
Must Read:
CBSE Class 12 Maths Unit Wise Marks Distribution 2024
| No. | Units | Marks |
| I | Relations and Functions | 08 |
| II | Algebra | 10 |
| III | Calculus | 35 |
| IV | Vectors and Three - Dimensional Geometry | 14 |
| V | Linear Programming | 05 |
| VI | Probability | 08 |
| | Total | 80 |
| | Internal Assessment | 20 |
Related:
CBSE Class 12 Maths Case Study Questions 2023
Question 1: Ramesh is elder brother of Suresh. Ramesh wants to help his younger brother Suresh to solve the following problems of integrals. Write the suitable substitution by which Ramesh can help him.
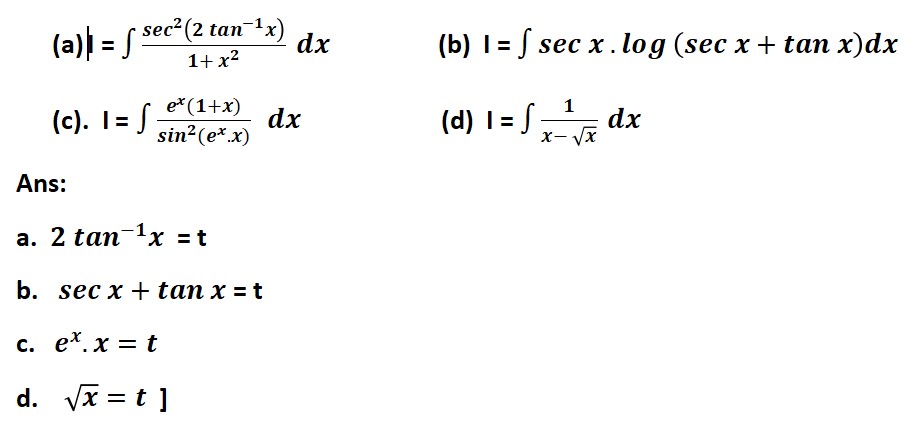
Question 2: Mr Shashi, who is an architect, designs a building for a small company. The design of window on the ground floor is proposed to be different than other floors. The window is in the shape of a rectangle which is surmounted by a semi-circular opening. This window is having a perimeter of 10 m as shown below :
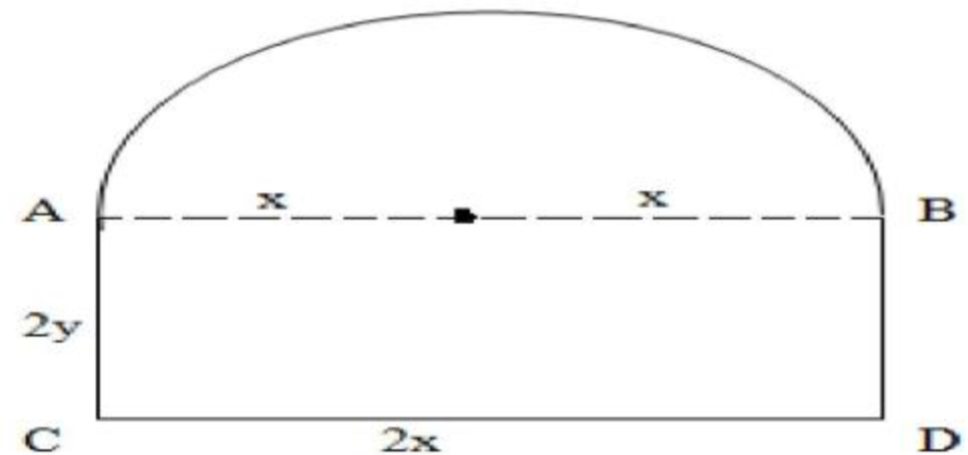
Based on the above information answer the following :
(i) If 2x and 2y represents the length and breadth of the rectangular portion of the windows, then the relation between the variables is:
(ii) The combined area (A) of the rectangular region and semi-circular region of the window expressed as a function of x is:
(iii) The maximum value of area A, of the whole window is
OR
The owner of this small company is interested in maximizing the area of the whole window so that maximum light input is possible.
For this to happen, the length of rectangular portion of the window should be
Answer:
(i) 4y = 10 - (2 + π)x
(ii) A = 10x - (2 + 12π)x2
(iii) 50/4 + π
OR
20/4 + π
Question 3: Read the following and answer the questions given below
The front gate of a building is in the shape of a trapezium as shown below. Its three sides other than base are of 10 m each. The height of the gate is h meter. On the basis of below figure, answer the following questions:

(i) Write the Area (A) of the gate in terms of .
(ii) Write the value of when Area (A) is maximum.
(iii) Write the value of h when Area (A) is maximum .
OR
Write the Maximum value of Area (A) .
Answer:
(i) (10 + x)√100 - x2
(ii) 5m
(iii) 5√3m OR 75√3/m.m2
Question 4: Read the following and answer the questions given below
Given three identical boxes 1st, 2nd and 3rd each containing two coins. In 1st box both coins are gold coins, in 2nd box both are silver coins and in 3rd box there is one gold and one silver coin. A person chooses a box at random and takes out a coin.
On the basis of above information, answer the following questions:
(i) What is the probability of choosing 1st box ?
(ii) What is the probability of getting gold coin from 3rd box ?
(iii) What is the total probability of drawing gold coin ?
OR
If drawn coin is of gold the probability that other coin in the box is also of gold?
Answer:
(i) 1/3
(ii) 1/2
(iii) 1/2 Or 2/3
Question 5: Read the following and answer the questions given below
Sand is pouring from a pipe at the rate of 12 cm3/ second the falling sand forms a cone on the ground in such a way that the height of the cone is always 1/6th of the radius of the base. Based on above information answer the following:
(i) Write the expression for volume in terms of height only.
(ii) What is the rate of Change of height, when height is 4 cm?
Answer:
i) 12πh3
(ii) 1/48 cm/s
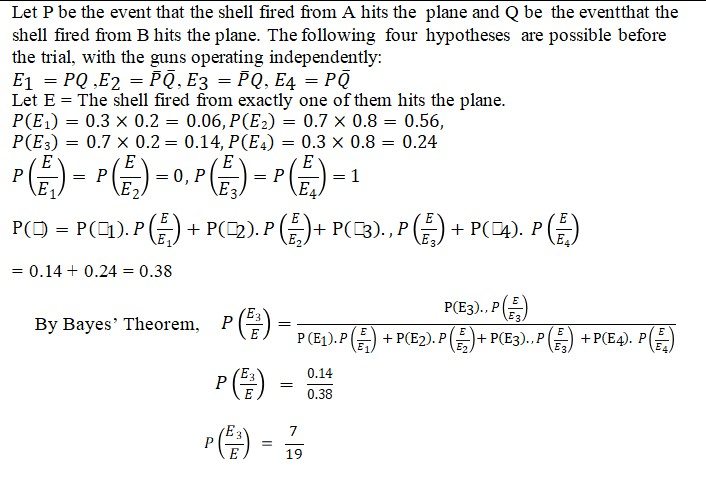
Question 6: There are two antiaircraft guns, named as A and B. The probabilities that the shell fired from them hits an airplane are 0.3 and 0.2 respectively. Both of them fired one shell at an airplane at the same time.
(i) What is the probability that the shell fired from exactly one of them hit the plane?
(ii) If it is known that the shell fired from exactly one of them hit the plane, then what is the probability that it was fired from B?
Answer:
Also Read:
Other Important Study Resources for CBSE Class 12 Exams
